3D Cube Software Rendering

Windows Source Files

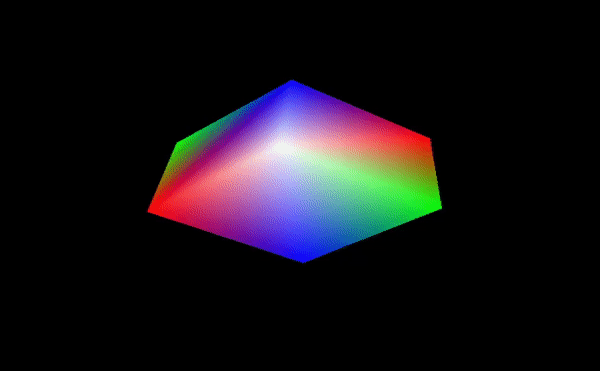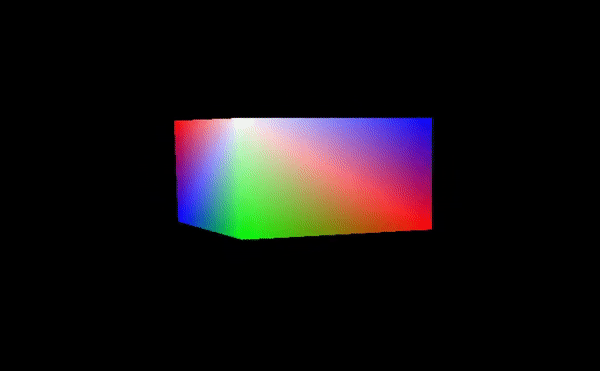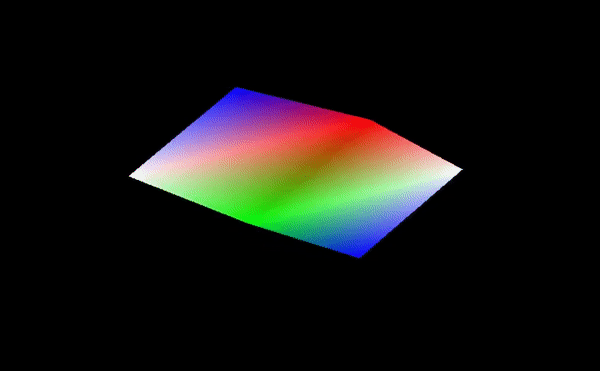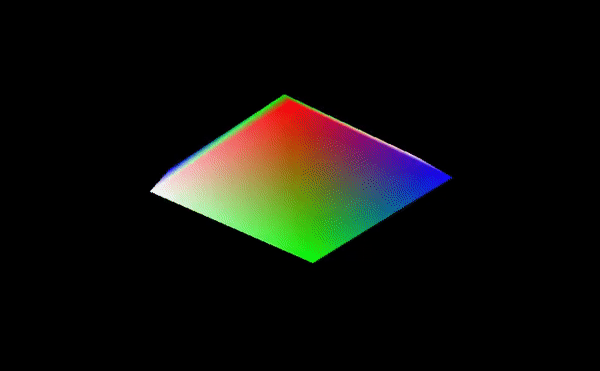
Create a 3D Cube from Scratch

When I say from scrach what I mean is that we are not calling any functions specifically to perform rendering functions from libraries such as Opengl or Direct3D. Instead on the Windows operating system we have a bitmap which we can change the color values each frame and that is it!

To learn more about 3D graphics I wanted to rasterize a single Cube. Before I could do that I needed to render a triangle.

Render a Triangle

  1. Perspective Project 3D points to 2D viewing Plane
    2. 				
					V3
					PerspectiveProject(V3 Point, V3 Camera)
					{
						V3 result={};
						result.X = ((Point.X+Camera.X) * Camera.Z)/(Camera.Z - Point.Z);
						result.Y = ((Point.Y+Camera.Y) * Camera.Z)/(Camera.Z - Point.Z);
						result.Z = Point.Z;
						return result;
					}
  2. Normalize coordiantes between [0,1]
  3. resize the coordiates to raster space by multipling by the width and height of the window.
  4. loop over the points that make up the Triangle
  5. If the point is inside the triangle fill it in with color
  6. the way to determine whether a point is inside the triangle is found using this equation
    7. 				
					float
					edgeFunction(V2 a,V2 b, V2 c)
					{
						return(((c.X - a.X)*(b.Y-a.Y))-((c.Y-a.Y)*(b.X-a.X))) ;
					}
					//p being the point we want to test in raster space
					//V0 being a Vertex of the triangle in raster space
					//V0 being a Vertex of the triangle in raster space
					float w2 =edgeFunction(V0,V1,p);
  7. that value returned by each call of the function,also represents the color value. this is known as Barycentric Coordinates
  8. Multiply the vertex color by the Barycentric coordiate value to determine the color

Rasterize the Cube

Once I was able to rasterize a single trianlge I had to For this project I created wanted to create a 3d Rasterizer

Rotation

Link
  1. When Rotating about an axis you change the other two dimensions, If you rotate on the x axis then the y and z coordiates undergo a translation.
  2. We are able to calculate a rotated position knowing only the
    • Rotation Point, the point we wish to rotate
    • Pivot Point, the point which we are rotating about
    • Angle of Rotation, the angle for each axis
  • If X is the axis of rotation
    • 				
				float deltaZ = rPoint.Z - PivotPoint.Z;
				float deltaY = rPoint.Y - PivotPoint.Y;
				float magnitude = sqrt((deltaZ*deltaZ)+(deltaY*deltaY));
				
				float existingAngle = atan2(deltaY,deltaZ);
				
				rPoint.Z = PivotPoint.Z + (magnitude*(cosf(AngleOfRotation.X+existingAngle)));
				rPoint.Y = PivotPoint.Y + (magnitude*(sinf(AngleOfRotation.X+existingAngle)));